
in this case the hipothesis is that s=1/2 and s=1/2+it are zeroes of the zeta function
an interesting view of the problem is if we abstract of the base in wich we "see" the numbers
 orthogonality
orthogonality ![{d \over dx} \left[ (1-x^2) {d \over dx} P(x) \right] = -\lambda P(x),](http://upload.wikimedia.org/math/b/9/3/b93b0519eba36d1149a5ad46e123f14d.png)
because det(L) = 1; the right hand side is easily computed as the product of all diagonal elements of U multiplied with the determinant of the permutation matrix P (which is +1 for an even permutation and is -1 for an odd permutation). This is more efficient than calculating the determinant of A because the determinant of a (upper or lower) triangular matrix is the product of its diagonal elements.
A small example:
Therefore
due the calculations are linnear
det(A-lambdasubi*I=C)=0->in a base B we obtain the diagonal matrix ,
B*C*B^-1=I
(2 0 0 0.................................0) ( )=(-(2^n)-1 n=0
(0 4 0 0..................................0) ---- (-2^n)-2=0
(----------------------------...............0) ... ...
C=((0 0 2^(a-1) 00..,00.....................0) X= (2^(n-1)-(2^n))=0=-1/2=lambdaN for all n
(0 0 3 0 0 --...............0)
( ----------------------------------------
(0 0 0 3^4 0 ....................0)
(0 0 0 0 0 3^b 0..0..00)
( ........................................ )
(0...... ...pi^k................0)
. (..................................................0)
(0....----------..........................pn^n) , det(C)=p(n+1)-1 even,pair number=2^a*3^b*5^c*pn^n ,
it conforms the system of equations: det(C-2^a*I)=0..det(C-3^b*I)=0
we also can use the conjecture of coldbach pi+pm=((p(n+1)-1))*(1/(p(n+1)-1)^n)=even
(1/(p(n+1)-1)^n)*(pi+pm)=((p(n+1)-1))->(1/(p(n+1)-1)^n)*(2^a*3^b*5^c*p(i-1)^(i-1)+2^a*3^b*5^c*p(m-1)^(m-1))
q,c,d
seeing at another perspective
(1 0 0 ) (0 0 1)
C=(2 0 0...) C-1=(0 0 1/2)
(0 4 0...) (0 1/4 0)
(0 0 8...) (1/8 0 0)
(......... ) ............
(0 0 0 2^a) (1/2^a 0 0)
Det[(C)tensor product x Ntimes x C x (D) .. Ntimes D x ... N x Ntimes x..xN]
= ((lambda1=-1=(1/(2*2^2*2^4*2^3*..2^N)^(2*2^2*2^4*2^3*..2^N))+1)*(lambda2=
-1=1/(3^2*3^3*3^4*..*3^N)^ (3^2*3^3*3^4*..*3^N))+1)*...*(lambdaN=
-1=1/(pN*pN^2*pN^3*..*P^N)^(pN*pN^2*pN^3*..*P^N))+1)=zeta(S)=0
=((p^S)-1)/p^S=
Det(C-1)*Det[(C-1)tensor product x (D-1)x..x(N-1)]*Det(1/C^t (tensor product=x) 1/D^t ) x 1/E^t x..1/(N-1)^t)*
Det(CxDx..xN)*(C^-1xD^-1x..xN^-1)=Ix..Ntimes..xI lambdai=1^N just need to apply in a recurrent form conjecture of coldback prime1+prime2=even so we also can use the conjecture of coldbach pi+pm=((p(n+1)-1))*(1/(p(n+1)-1)^n)=even develop and obtain final solution or last fermat theorem pi^n+pj^n=pk^n
C=(C/2-2*lambda*IxIxI..I) ->lambdasubi=2^i
D=(3 0 0...)
(0 9 0...)
(0 0 27..)
.....
(0 0 0 3^b)
D=D/3-3lambdaIxIxI..I .>lambdasubj=3^j
E=(5 0 0...0)
(0 0 25..0)
...........
(0 0 0 5^c)
....
N=(pn 0 0 ..0)
(0 pn^2 0..0)
. ................
(0 0 0 pn^z)
|((C tensor product D tensor product E ... tensor product N)-(lambda=(2^a*3^b*5^c*p(i-1)^(i-1) ( I tensor product I ... tensor product I) ....|=0->lambdasubi=pi=
PA=LU->PA=I*B
B*C=I*B->C=B^-1*B=B^t*B/det|B|->det(C) normalized= p(n+1)-1=(2^a/(2^a*3^b*5^c*pn^n))*(3^b/(2^a*3^b*5^c*pn^n))*..*((pn^n/(2^a*3^b*5^c*pn^n))=
(p(n+1)-1)=((p(n+1)-1))*(1/(p(n+1)-1)^n)
det(A-lambdasubi*I=C)=0
Method to obtain prime numbers:
det|A'-lambdasubi*I|=0
A'=(1 111111111111111111..
(2 4 8 16 32 64......
(3 9 27 71..........................
(4 16 24...
(5 25 125..
(6 36 36^2......
(7 14 98 .....
(8 64.. mxm
....
A=(1 0 0 0 0 0 00 0 0 0 0..
(2 4 0 0 0 0 00 0 0 0 ......
(3 9 27 0 0 0 0 0 0 0 0..........................
(4 16 32 64 0 0 0 00 0...
(5 25 125 625 5^6 0 0 0..
(6 36 36^2 36^4 36^5 36^6 36^7......
( ....................................... mxm
det|A-lambdasubi*I|=0
hacemos combinaciones lineales de las filas que nos da la misma det de la matriz
M'=(
(fila1=(pot2)A0,0..n+A1,0..n+A3,0..n+A7,0..n+A(8+64-1),0..n...
(fila2(pot3)A0,0..n+A2,0..n+A8,0..n+A16,0..n+A24,0..n+......
(fila3=(pot5).A0,0..n+A4,0..n..
tenemos que , we have that
fila1=2^m=Am,0=pow(2m)(A0,0),A7,0=pow(2)(A3,0);A3,0=pow(2)(A1,0=2*A0,0))
fila2=3^m=Am,0=pow(3m)(A0,0),A8,0=pow(3)(A4,0);A3,0=pow(3)(A2,0=3*A0,0))
fila3=5^m=Am,0=pow(5m)(A0,0),A100,0=pow(5)(A20,0);A20,0=pow(3)(A4,0=3*A0,0))
luego then
fila1=2^m=Am,0;xm*pow(2m)(A0,0)+..+x2*A7,0+x1*pow(2)(A3,0);A3,0+x0*pow(2)(A1,0=2*A0,0))=0
fila2=3^m=Am,0;xm*pow(3m)(A0,0)+..+x2*A8,0+x1*pow(3)(A4,0);A3,0+x0*pow(3)(A2,0=3*A0,0))=0
fila3=5^m=Am,0;xm*pow(5m)(A0,0)+..+x2*A100,0+x1*pow(5)(A20,0);A20,0+x0*pow(5)(A4,0=3*A0,0))=0
luego then
det|fila1=2^m=(Am,0)pow(2m)-lambdam.. ,,A7,0 ...pow(2)(A3,0);A3,0.... ... pow(2)(A1,0=2*A0,0))=0
|fila2=3^m=Am,0;pow(3m)(A0,0).......x2*A8,0;pow(3)(A4,0)-lambda1;A3,0*pow(3)(A2,0=3*A0,0))=0
|fila3=5^m=Am,0;xm*pow(5m)(A0,0)+..+x2*A100,0+x1*pow(5)(A20,0);A20,0+pow(5)(A4,0=3*A0,0))-lambda0=0
(1-lamdba1)*(2-lambda2)*(3-lambda3)*..*(p-lambdap)=0
(1-lamdba1)^-S*(2-lambda2)^-S*(3-lambda3)^-S*..*(p-lambdap)^-S=0^-S=0
that is the case the matrix M' has 2 rows repeated -S/2=a, -S/3=b,.. integer that gives out trivial zeroes
for det(A)=0->prod(Ai,i)*...*((Ai-m,i-m)^-S=Aii^(-m*S)) S=-2,-3,-5,-7..prime gives out trivial zeroes
so probe the hipothesis of riemman results trivial:
((Ai-m,i-m)^-S=Aii^(m/2)) that is the definition of the trigonal matrix S=-1/2 S=-1/2+ib not altering the condition of det(A)=0
pk=m*n if pk|basepk=1->m*n|base(m*n)=1->m|basen=1/(n|basem)
changing to base 2 binary and eliminating 0=/2 in base 3 /3 etc
a power base n*n=20->base10|n*n
Q where Q is a prime number
2=(Q-1) mod ((Q-1)/2))
Q mod(Q-1)=1=((Q-1) mod ((Q-1)/2)))-1
(Q-1) mod(Q-2)=1
(Q-2) mod (Q-3)=1
Q mod(Q-1=mod(Q-2=mod(Q-3=...(mod 1)...))=1
they are infinite prime generators so:
the solution of hypothesis of riemman thus is trivial just
use (pk-1) mod (((pk-1)/2)))=(pk-1)/../2 mod ((pk-1)/../2)/2)
with
etha(s)=prod|n=0,n=inf 1/n^s=(1+1/2^S+1/4^S+1/16^S+...)*(1+1/3^S+..)*
with
The properties that make this relation a congruence relation (respecting addition, subtraction, and multiplication) are the following.
If
and
then:
It should be noted that the above two properties would still hold if the theory were expanded to include allreal numbers, that is if  were not necessarily all integers. The next property, however, would fail if these variables were not all integers:
were not necessarily all integers. The next property, however, would fail if these variables were not all integers:
 were not necessarily all integers. The next property, however, would fail if these variables were not all integers:
were not necessarily all integers. The next property, however, would fail if these variables were not all integers:etha(s)=prod|n=0,n=inf 1/n^s=(1+1/2^S+1/4^S+1/16^S+...)*(1+1/3^S+..)*
*(1+1/pk^S+1/pk^2S+...+1/pk^(N*S))=0
thats it!!
thats it!!
also the zero of a product is the product of zeroes,
Mathematician wins Shaw Prize for prime numbers, symmetry unification
September 12, 2007
Herchel Smith Professor of Mathematics Richard Taylor has been awarded the Shaw Prize in Mathematical Sciences for work that unified the diverse fields of prime numbers and symmetry.
Taylor shares the prize with Princeton Professor Robert Langlands, who initiated work in the field that was subsequently built upon by Taylor.
The honor is awarded by the Hong Kong-based Shaw Prize Foundation. The Shaw Prizes are given in three fields: astronomy, life sciences and medicine, and mathematical sciences. They are intended to recognize individuals currently active in their fields who have achieved “distinguished and significant advances, who have made outstanding contributions in culture and the arts, or who in other domains have achieved excellence,” according to the prize’s Web site.
Each prize comes with a $1 million award, which Taylor and Langlands will share.
The prizes will be awarded Tuesday (Sept. 11) during a ceremony in Hong Kong. Taylor will deliver a 45-minute Shaw Lecture on Wednesday.
Taylor said he learned of the prize in June when he checked his e-mail before going to bed. He said he knew he had been nominated for the prize, but thought it would be awarded to someone who was further along in his or her career.
Taylor’s work examines the properties of prime numbers — those numbers, such as 3, 5, 7, and 11, that are divisible only by themselves and 1. His work seeks to understand why they appear where they do among other numbers.
The explanation, it turns out, involves an entirely different field of mathematics — geometry, specifically the symmetry of curved spaces.
“You wouldn’t have thought that prime numbers, which is counting, has anything to do with geometry,” Taylor said.
The theories connecting the two fields are quite powerful, Taylor said, and even small advances have helped answer old mathematical problems that don’t appear to be related.
One such problem, Taylor said, was Fermat’s Last Theorem, a mathematical problem that had defied solution for 357 years. Andrew Wiles of Princeton first proposed a proof of the theorem in 1993, but an error was found. He called on Taylor, a former student of his, and together they completed the proof in 1994.
Taylor’s work also led to the solution of another old mathematical problem: the 40-year old Sato-Tate conjecture, in 2006, the work Taylor said may have led to his receiving the prize.
Taylor’s future work will continue to explore the field.
“We’re a long way from finishing,” he said.
Taylor received his doctorate from Princeton in 1988, coming to Harvard in 1996. He was named the Herchel Smith Professor of Mathematics in 2002. He has received numerous awards and honors, including the Fermat Prize for Mathematics in 2001, the Cole Prize for Number Theory in 2002, and the Dannie Heinemann Prize from the Gottingen Academy of Sciences in 2005.
Source: Harvard University
Ill give a clue for developing new solutions
just equally etha(s)=0 at each expression or combination and develope functons in a powers or multiplication of primes way =Cn with adjusted polinomials (a+1/b+1/c)=0 * (..) * (...) etc
use your imagination!!
I also accept a non already proof theorem or prime conjecture as a valid solution of the shared million prize as conjecture of goldback see above,proven.
Prime number
From Wikipedia, the free encyclopedia
"Prime" redirects here. For other uses, see Prime (disambiguation).
A prime number (or a prime) is a natural number that has exactly two distinct natural number divisors: 1 and itself. The smallest twenty-five prime numbers (all the prime numbers under 100) are:
An infinitude of prime numbers exists, as demonstrated by Euclid around 300 BC,[2] although the density of prime numbers within natural numbers is 0. The number 1 is by definition not a prime number. The fundamental theorem of arithmetic establishes the central role of primes in number theory: any nonzero natural number n can be factored into primes, written as a product of primes or powers of different primes (including the empty product of factors for 1). Moreover, this factorization is unique except for a possible reordering of the factors.
The property of being prime is called primality. Verifying the primality of a given number n can be done by trial division. The simplest trial division method tests whether n is a multiple of an integer m between 2 and 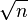 . If n is a multiple of any of these integers then it is a composite number, and so not prime; if it is not a multiple of any of these integers then it is prime. As this method requires up to
. If n is a multiple of any of these integers then it is a composite number, and so not prime; if it is not a multiple of any of these integers then it is prime. As this method requires up to 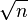 trial divisions, it is only suitable for relatively small values of n. More sophisticated algorithms, which are much more efficient than trial division, have been devised to test the primality of large numbers.
trial divisions, it is only suitable for relatively small values of n. More sophisticated algorithms, which are much more efficient than trial division, have been devised to test the primality of large numbers.
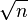 . If n is a multiple of any of these integers then it is a composite number, and so not prime; if it is not a multiple of any of these integers then it is prime. As this method requires up to
. If n is a multiple of any of these integers then it is a composite number, and so not prime; if it is not a multiple of any of these integers then it is prime. As this method requires up to 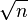 trial divisions, it is only suitable for relatively small values of n. More sophisticated algorithms, which are much more efficient than trial division, have been devised to test the primality of large numbers.
trial divisions, it is only suitable for relatively small values of n. More sophisticated algorithms, which are much more efficient than trial division, have been devised to test the primality of large numbers.
There is no known useful formula that yields all of the prime numbers and no composites. However, the distribution of primes, that is to say, the statistical behaviour of primes in the large can be modeled. The first result in that direction is the prime number theorem which says that the probability that a given, randomly chosen number n is prime is inversely proportional to its number of digits, or the logarithm of n. This statement has been proven since the end of the 19th century. The unproven Riemann hypothesis dating from 1859 implies a refined statement concerning the distribution of primes.
Despite being intensely studied, there remain some open questions around prime numbers which can be stated simply. For example, Goldbach's conjecture, which asserts that any even natural number bigger than two is the sum of two primes, and thetwin prime conjecture, which says that there are infinitely many twin primes (pairs of primes whose difference is two), have been unresolved for more than a century, notwithstanding the simplicity of their statements.
Prime numbers give rise to various generalizations in other mathematical domains, mainly algebra, notably the notion of prime ideals.
Primes are applied in several routines in information technology, such as public-key cryptography, which makes use of the difficulty of factoring large numbers into their prime factors. Searching for big primes, often using distributed computing, has stimulated studying special types of primes, chiefly Mersenne primes whose primality is comparably quick to decide. As of 2010, the largest known prime number has about 13 million decimal digits.[3]
Contents[hide] |
[edit]Prime numbers and the fundamental theorem of arithmetic
Main article: Fundamental theorem of arithmetic
A natural number is called a prime or a prime number if it has exactly two distinct natural number divisors. Natural numbers greater than 1 that are not prime are called composite. Therefore, 1 is not prime, since it has only one divisor, namely 1. However, 2 and 3 are prime, since they have exactly two divisors, namely 1 and 2, and 1 and 3, respectively. Next, 4, is composite, since it has 3 divisors: 1, 2, and 4.
Using symbols, a number n > 1 is prime if it cannot be written as a product of two integers a and b, both of which are larger than 1:
- n = a · b.
The crucial importance of prime numbers to number theory and mathematics in general stems from the fundamental theorem of arithmetic which states that every positive integer larger than 1 can be written as a product of one or more primes in a way which is unique except possibly for the order of the prime factors. Primes can thus be considered the “basic building blocks” of the natural numbers. For example, we can write:
23244 = 2 · 2 · 3 · 13 · 149 = 22 · 3 · 13 · 149. (22 denotes the square or second power of 2.)
As in this example, the same prime factor may occur multiple times. A decomposition:
- n = p1 · p2 · ... · pt
of a number n into (finitely many) prime factors p1, p2, ... to pt is called prime factorization of n. The fundamental theorem of arithmetic can be rephrased so as to say that any factorization into primes will be identical except for the order of the factors. So, albeit there are many prime factorization algorithms to do this in practice for larger numbers, they all have to yield the same result.
The set of all primes is often denoted P.
[edit]Examples and first properties
The only even prime number is 2, since any larger even number is divisible by 2. Therefore, the term odd prime refers to any prime number greater than 2.
The image at the right shows a graphical way to show that 12 is not prime. More generally, all prime numbers except 2 and 5, written in the usual decimal system, end in 1, 3, 7 or 9, since numbers ending in 0, 2, 4, 6 or 8 are multiples of 2 and numbers ending in 0 or 5 are multiples of 5. Similarly, all prime numbers above 3 are of the form 6n − 1 or 6n + 1, because all other numbers are divisible by 2 or 3. Generalizing this, all prime numbers above q are of form q#·n + m, where 0 < m < q#, and m has no prime factor ≤ q.
If p is a prime number and p divides a product ab of integers, then p divides a or p divides b. This proposition is known as Euclid's lemma. It is used in some proofs of the uniqueness of prime factorizations.
[edit]Primality of one
One of the primary reasons to exclude 1 from the set of prime numbers is the fundamental theorem of arithmetic, which says that every positive integer x can be uniquely written as a product of primes. When x is itself prime, this factorization has only one prime (x itself) in it, and when x = 1 the factorization is the empty product. But if 1 were admitted as a prime, then any integer could be factored in an infinite number of ways. For example, in this case the number 3 could be factored as 1k · 3 = 3 for any integer k.
More generally, in unique factorization domains, every non-zero element is a unique product of prime elements and a unit. The factorization would not be unique if products of units were allowed.
Until the 19th century, most mathematicians considered the number 1 a prime, the definition being just that a prime is divisible only by 1 and itself but not requiring a specific number of distinct divisors. There is still a large body of mathematical work that is valid despite labeling 1 a prime, such as the work of Stern and Zeisel. Derrick Norman Lehmer's list of primes up to 10,006,721, reprinted as late as 1956,[4] started with 1 as its first prime.[5] Henri Lebesgue is said to be the last professional mathematician to call 1 prime.[6] The change in label occurred so that the fundamental theorem of arithmetic, as stated, is valid, i.e., “each number has a unique factorization into primes.”[7][8] Furthermore, the prime numbers have several properties that the number 1 lacks, such as the relationship of the number to its corresponding value of Euler's totient function or the sum of divisors function.[9]
[edit]History
There are hints in the surviving records of the ancient Egyptians that they had some knowledge of prime numbers: the Egyptian fraction expansions in the Rhind papyrus, for instance, have quite different forms for primes and for composites. However, the earliest surviving records of the explicit study of prime numbers come from the Ancient Greeks. Euclid's Elements (circa 300 BC) contain important theorems about primes, including the infinitude of primes and the fundamental theorem of arithmetic. Euclid also showed how to construct a perfect number from a Mersenne prime. TheSieve of Eratosthenes, attributed to Eratosthenes, is a simple method to compute primes, although the large primes found today with computers are not generated this way.
After the Greeks, little happened with the study of prime numbers until the 17th century. In 1640 Pierre de Fermat stated (without proof) Fermat's little theorem (later proved by Leibniz and Euler). A special case of Fermat's theorem may have been known much earlier by the Chinese. Fermat conjectured that all numbers of the form 22n + 1 are prime (they are called Fermat numbers) and he verified this up to n = 4 (or 216 + 1). However, the very next Fermat number 232 + 1 is composite (one of its prime factors is 641), as Euler discovered later, and in fact no further Fermat numbers are known to be prime. The French monk Marin Mersenne looked at primes of the form 2p − 1, with p a prime. They are called Mersenne primes in his honor.
Euler's work in number theory included many results about primes. He showed the infinite series 1/2 + 1/3 + 1/5 + 1/7 + 1/11 + … is divergent. In 1747 he showed that the even perfect numbers are precisely the integers of the form 2p−1(2p − 1), where the second factor is a Mersenne prime.
At the start of the 19th century, Legendre and Gauss independently conjectured that as x tends to infinity, the number of primes up to x is asymptotic to x/ln(x), where ln(x) is the natural logarithm ofx. Ideas of Riemann in his 1859 paper on the zeta-function sketched a program which would lead to a proof of the prime number theorem. This outline was completed by Hadamard and de la Vallée Poussin, who independently proved the prime number theorem in 1896.
Proving a number is prime is not done (for large numbers) by trial division. Many mathematicians have worked on primality tests for large numbers, often restricted to specific number forms. This includes Pépin's test for Fermat numbers (1877), Proth's theorem (around 1878), the Lucas–Lehmer primality test (originated 1856),[10] and the generalized Lucas primality test. More recent algorithms like APRT-CL, ECPP, and AKS work on arbitrary numbers but remain much slower.
For a long time, prime numbers were thought to have extremely limited application outside of pure mathematics;[citation needed] this changed in the 1970s when the concepts of public-key cryptography were invented, in which prime numbers formed the basis of the first algorithms such as the RSA cryptosystem algorithm.
Since 1951 all the largest known primes have been found by computers. The search for ever larger primes has generated interest outside mathematical circles. The Great Internet Mersenne Prime Search and other distributed computing projects to find large primes have become popular in the last ten to fifteen years, while mathematicians continue to struggle with the theory of primes.
[edit]The number of prime numbers
Main article: Euclid's theorem
There are infinitely many prime numbers. The oldest known proof for this statement, sometimes referred to as Euclid's theorem, is attributed to the Greek mathematician Euclid. Euclid states the result as "there are more than any given [finite] number of primes", and his proof is essentially the following:
Consider any finite set of primes. Multiply all of them together and add 1 (see Euclid number). The resulting number is not divisible by any of the primes in the finite set we considered, because dividing by any of these would give a remainder of 1. Because all non-prime numbers can be decomposed into a product of underlying primes, then either this resultant number is prime itself, or there is a prime number or prime numbers which the resultant number could be decomposed into but are not in the original finite set of primes. Either way, there is at least one more prime that was not in the finite set we started with. This argument applies no matter what finite set we began with. So there are more primes than any given finite number. (Euclid, Elements: Book IX, Proposition 20)
This previous argument explains why the product P of finitely many primes plus 1 must be divisible by some prime (possibly itself) not among those finitely many primes.
The proof is sometimes phrased in a way that falsely leads some readers to think that P + 1 must itself be prime, and think that Euclid's proof says the prime product plus 1 is always prime. This confusion arises when the proof is presented as a proof by contradiction and P is assumed to be the product of the members of a finite set containing all primes. Then it is asserted that if P + 1 is not divisible by any members of that set, then it is not divisible by any primes and "is therefore itself prime" (quoting G. H. Hardy[11]). This sometimes leads readers to conclude mistakenly that if P is the product of the first n primes then P + 1 is prime. That conclusion relies on a hypothesis later proved false, and so cannot be considered proved. The smallest counterexample with composite P + 1 is
- 2 × 3 × 5 × 7 × 11 × 13 + 1 = 30,031 = 59 × 509 (both primes).
Many more proofs of the infinitude of primes are known. Adding the reciprocals of all primes together results in a divergent infinite series:
The proof of that statement is due to Euler. More precisely, if S(x) denotes the sum of the reciprocals of all prime numbers p with p ≤ x, then
- S(x) = ln ln x + O(1) for x → ∞.
Another proof based on Fermat numbers was given by Goldbach.[12] Kummer's is particularly elegant[13] and Harry Fürstenberg provides one using general topology.[14]
Not only are there infinitely many primes, Dirichlet's theorem on arithmetic progressions asserts that in every arithmetic progression a, a + q, a + 2q, a + 3q, … where the positive integers a and q are coprime, there are infinitely many primes. The recentGreen–Tao theorem shows that there are arbitrarily long arithmetic progressions consisting of primes.[15]
[edit]Verifying primality
Main article: Primality test
To use primes requires verifying whether a given number n is prime or not. There are several ways to achieve this. A sieve is an algorithm that yields all primes up to a given limit. The oldest such sieve is the sieve of Eratosthenes (see above), useful for relatively small primes. The modern sieve of Atkin is more complicated, but faster when properly optimized. Before the advent of computers, lists of primes up to bounds like 107 were also used.[16]
In practice, one often wants to check whether a given number is prime, rather than generate a list of primes as the two mentioned sieve algorithms do. The most basic method to do this, known as trial division, works as follows: given a number n, one divides n by all numbers m less than or equal to the square root of that number. If any of the divisions come out as an integer, then the original number is not a prime. Otherwise, it is a prime. Actually it suffices to do these trial divisions for those m that are prime, only. While an easy algorithm, it quickly becomes impractical for testing large integers because the number of possible factors grows too rapidly as the number-to-be-tested increases: According to the prime number theorem expounded below, the number of prime numbers less than n is near n / (ln (n) − 1). So, to check n for primality the largest prime factor needed is just less than 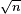 , and so the number of such prime factor candidates would be close to
, and so the number of such prime factor candidates would be close to  . This increases ever more slowly with n, but, because there is interest in large values for n, the count is large also: for n = 10 20 it is 450 million.
. This increases ever more slowly with n, but, because there is interest in large values for n, the count is large also: for n = 10 20 it is 450 million.
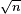 , and so the number of such prime factor candidates would be close to
, and so the number of such prime factor candidates would be close to  . This increases ever more slowly with n, but, because there is interest in large values for n, the count is large also: for n = 10 20 it is 450 million.
. This increases ever more slowly with n, but, because there is interest in large values for n, the count is large also: for n = 10 20 it is 450 million.
Modern primality test algorithms can be divided into two main classes, deterministic and probabilistic (or "Monte Carlo") algorithms. Probabilistic algorithms may report a composite number as a prime, but certainly do not identify primes as composite numbers; deterministic algorithms on the other hand do not have the possibility of such erring. The interest of probabilistic algorithms lies in the fact that they are often quicker than deterministic ones; in addition for most such algorithms the probability of erroneously identifying a composite number as prime is known. They typically pick a random number a called a "witness" and check some formula involving the witness and the potential prime n. After several iterations, they declare n to be "definitely composite" or "probably prime". For example, Fermat's primality test relies on Fermat's little theorem (see above). Thus, if
- ap − 1 (mod p)
is unequal to 1, p is definitely composite. However, p may be composite even if ap − 1 = 1 (mod p) for all witnesses a, namely when p is a Carmichael number. In general, composite numbers that will be declared probably prime no matter what witness is chosen are called pseudoprimes for the respective test. However, the most popular probabilistic tests do not suffer from this drawback. The following table compares some primality tests. The running time is given in terms of n, the number to be tested and, for probabilistic algorithms, the number k of tests performed.
| Test | Developed in | Deterministic | Running time | Notes |
|---|---|---|---|---|
| AKS primality test | 2002 | Yes | O(log6+ε(n)) | |
| Fermat primality test | No | O(k · log2n · log log n · log log log n) | fails for Carmichael numbers | |
| Lucas primality test | Yes | requires factorization of n − 1 | ||
| Solovay–Strassen primality test | 1977 | No, error probability 2−k | O(k·log3 n) | |
| Miller–Rabin primality test | 1980 | No, error probability 4−k | O(k · log2 n · log log n · log log log n) | |
| Elliptic curve primality proving | 1977 | No | O(log5+ε(n)) | heuristic running time |
[edit]Special types of primes
Further information: List of prime numbers
There are many particular types of primes, for example qualified by various formulae, or by considering its decimal digits. Primes of the form 2p − 1, where p is a prime number, are known as Mersenne primes. Their importance lies in the fact that there are comparatively quick algorithms testing primality for Mersenne primes.
Primes of the form 22k + 1 are known as Fermat primes; a regular n-gon is constructible using straightedge and compass if and only if
- n = 2i · m
where m is a product of any number of distinct Fermat primes and i is any natural number, including zero. Only five Fermat primes are known: 3, 5, 17, 257, and 65,537. Prime numbers p where 2p + 1 is also prime are known as Sophie Germain primes. A prime p is called primorial or prime-factorial if it has the form
- p = n# ± 1
for some number n, where n# stands for the product 2 · 3 · 5 · 7 · … of all the primes ≤ n. A prime is called factorial if it is of the form n! ± 1. It is not known whether there are infinitely many primorial or factorial primes.
[edit]Location of the largest known prime
Main articles: Largest known prime and Mersenne prime
Since the dawn of electronic computers the largest known prime has almost always been a Mersenne prime because there exists a particularly fast primality test for numbers of this form, the Lucas–Lehmer primality test. The following table gives the largest known primes of the mentioned types.
| Prime | Number of decimal digits | Type | Date | Found by |
|---|---|---|---|---|
| 243,112,609 − 1 | 12,978,189 | Mersenne prime | August 23, 2008 | Great Internet Mersenne Prime Search |
| 19,249 × 213,018,586 + 1 | 3,918,990 | not a Mersenne prime (Proth number) | March 26, 2007 | Seventeen or Bust |
| 94550! − 1 | 429,390 | factorial prime | October 2010 | Domanov, PrimeGrid[17] |
| 392113# + 1 | 169,966 | primorial prime | 2001 | Heuer[18] |
| 65516468355 × 2333333 ± 1 | 100,355 | twin primes | 2009 | Twin prime search[19] |
Some of the largest primes not known to have any particular form (that is, no simple formula such as that of Mersenne primes) have been found by taking a piece of semi-random binary data, converting it to a number n, multiplying it by 256k for some positive integer k, and searching for possible primes within the interval [256kn + 1, 256k(n + 1) − 1].
The Electronic Frontier Foundation offered a US$100,000 prize to the first discoverers of a prime with at least 10 million digits. On October 22, 2009, the prize was awarded to the Great Internet Mersenne Prime Search (GIMPS) for discovering the 45th known Mersenne prime, which is 243,112,609 − 1. The UCLA mathematics department owns the computer on which the discovery was made and received half of the prize money, with the remainder going to charity and future research.[20] The EFF also offers $150,000 and $250,000 for 100 million digits and 1 billion digits, respectively.[21]
[edit]Generating prime numbers
Main article: Formula for primes
There is no known formula for primes which is more efficient at finding primes than the methods mentioned above.
There is a set of Diophantine equations in 9 variables and one parameter with the following property: the parameter is prime if and only if the resulting system of equations has a solution over the natural numbers. This can be used to obtain a single formula with the property that all its positive values are prime.
There is no polynomial, even in several variables, that takes only prime values. However, there are polynomials in several variables, whose positive values (as the variables take all positive integer values) are exactly the primes (for an example, see formula for primes).
Another formula is based on Wilson's theorem mentioned above, and generates the number 2 many times and all other primes exactly once. There are other similar formulas which also produce primes.
[edit]Distribution
Further information: Prime number theorem
Given the fact that there is an infinity of primes, it is natural to seek for patterns or irregularities in the distribution of primes. The problem of modeling the distribution of prime numbers is a popular subject of investigation for number theorists. The occurrence of individual prime numbers among the natural numbers is (so far) unpredictable, even though there are laws (such as the prime number theorem and Bertrand's postulate) that govern their average distribution. Leonhard Euler commented
Mathematicians have tried in vain to this day to discover some order in the sequence of prime numbers, and we have reason to believe that it is a mystery into which the mind will never penetrate.[22]
In a 1975 lecture, Don Zagier commented
There are two facts about the distribution of prime numbers of which I hope to convince you so overwhelmingly that they will be permanently engraved in your hearts. The first is that, despite their simple definition and role as the building blocks of the natural numbers, the prime numbers grow like weeds among the natural numbers, seeming to obey no other law than that of chance, and nobody can predict where the next one will sprout. The second fact is even more astonishing, for it states just the opposite: that the prime numbers exhibit stunning regularity, that there are laws governing their behavior, and that they obey these laws with almost military precision.[23]
Euler noted that the function
- n2 + n + 41
gives prime numbers[24] for n < 40 (but not necessarily so for bigger n),[25] a remarkable fact leading into deep algebraic number theory, more specifically Heegner numbers. The Ulam spiral depicts all natural numbers in a spiral-like way. Surprisingly, prime numbers cluster on certain diagonals and not others.
[edit]Number of prime numbers below a given number
Main article: Prime counting function
The prime-counting function π(n) is defined as the number of primes up to n. For example π(11) = 5, since there are five primes less than or equal to 11. There are known algorithms to compute exact values of π(n) faster than it would be possible to compute each prime up to n. Values as large as π(1020) can be calculated quickly and accurately with modern computers.
For larger values of n, beyond the reach of modern equipment, the prime number theorem provides an estimate: π(n) is approximately n/ln(n). In other words, as n gets very large, the likelihood that a number less thann is prime is inversely proportional to the number of digits in n. Even better estimates are known; see for example Prime number theorem#The prime-counting function in terms of the logarithmic integral.
If n is a positive integer greater than 1, then there is always a prime number p with n < p < 2n (Bertrand's postulate).
[edit]Gaps between primes
Main article: Prime gap
A sequence of consecutive integers none of which is prime constitutes a prime gap. There are arbitrarily long prime gaps: for any natural number n larger than 1, the sequence (for the notation n! read factorial)
- n! + 2, n! + 3, …, n! + n
is a sequence of n − 1 consecutive composite integers, since
- n! + m = m · (n!/m + 1) = m · [(1 · 2 · … · (m − 1) · (m + 1) … n) + 1]
is composite for any 2 ≤ m ≤ n. On the other hand, the gaps get arbitrarily small in proportion to the primes: the quotient
- (pi + 1 − pi) / pi,
where pi denotes the ith prime number (i.e., p1 = 2, p2 = 3, etc.), approaches zero as i approaches infinity.
[edit]Open questions
[edit]The Riemann hypothesis
Main article: Riemann hypothesis
To state the Riemann hypothesis, one of the oldest, yet still unproven, mathematical conjectures, it is necessary to understand the Riemann zeta function (s is a complex number with real part bigger than 1)
The second equality is a consequence of the fundamental theorem of arithmetics, and shows that the zeta function is deeply connected with prime numbers. For example, the fact (see above) that there are infinitely many primes can be read off from the divergence of the harmonic series:
If there were a finite number of primes then ζ(1) would have a finite value - but instead we know that the Riemann zeta function has a simple pole at 1.
Another example of the richness of the zeta function and a glimpse of modern algebraic number theory is the following identity (Basel problem), due to Euler,
Riemann's hypothesis is concerned with the zeroes of the ζ-function (i.e., s such that ζ(s) = 0). The connection to prime numbers is that it essentially says that the primes are as regularly distributed as possible. From a physical viewpoint, it roughly states that the irregularity in the distribution of primes only comes from random noise. From a mathematical viewpoint, it roughly states that the asymptotic distribution of primes (about 1/ log x of numbers less than x are primes, the prime number theorem) also holds for much shorter intervals of length about the square root of x (for intervals near x). This hypothesis is generally believed to be correct. In particular, the simplest assumption is that primes should have no significant irregularities without good reason.
[edit]Other conjectures
Further information: Category:Conjectures about prime numbers
Besides the Riemann hypothesis, there are many more conjectures about prime numbers, many of which are old: for example, all four of Landau's problems from 1912 (the Goldbach, twin prime, Legendre conjecture and conjecture about n2+1 primes) are still unsolved.
Many conjectures deal with the question whether an infinity of prime numbers subject to certain constraints exists. It is conjectured that there are infinitely many Fibonacci primes[26] and infinitely many Mersenne primes, but not Fermat primes.[27] It is not known whether or not there are an infinite number of prime Euclid numbers.
A number of conjectures concern aspects of the distribution of primes. It is conjectured that there are infinitely many twin primes, pairs of primes with difference 2 (twin prime conjecture). Polignac's conjecture is a strengthening of that conjecture, it states that for every positive integer n, there are infinitely many pairs of consecutive primes which differ by 2n. It is conjectured there are infinitely many primes of the form n2 + 1.[28] These conjectures are special cases of the broad Schinzel's hypothesis H.[citation needed] Brocard's conjecture says that there are always at least four primes between the squares of consecutive primes greater than 2. Legendre's conjecture states that there is a prime number between n2 and (n + 1)2 for every positive integer n. It is implied by the stronger Cramér's conjecture.
Other conjectures relate the additive aspects of numbers with prime numbers: Goldbach's conjecture asserts that every even integer greater than 2 can be written as a sum of two primes, while the weak version states that every odd integer greater than 5 can be written as a sum of three primes.
[edit]Applications
For a long time, number theory in general, and the study of prime numbers in particular, was seen as the canonical example of pure mathematics, with no applications outside of the self-interest of studying the topic. In particular, number theorists such asBritish mathematician G. H. Hardy prided themselves on doing work that had absolutely no military significance.[29] However, this vision was shattered in the 1970s, when it was publicly announced that prime numbers could be used as the basis for the creation of public key cryptography algorithms. Prime numbers are also used for hash tables and pseudorandom number generators.
Some rotor machines were designed with a different number of pins on each rotor, with the number of pins on any one rotor either prime, or coprime to the number of pins on any other rotor. This helped generate the full cycle of possible rotor positions before repeating any position.
The International Standard Book Numbers work with a check digit, which exploits the fact that 11 is a prime.
[edit]Arithmetic modulo a prime p
Main article: Modular arithmetic
Modular arithmetic is a modification of usual arithmetic, by doing all calculations "modulo" a fixed number n. All calculations of modular arithmetic take place in the finite set
- {0, 1, 2, ..., n − 1}.
Calculating modulo n means that sums, differences and products are calculated as usual, but then only the remainder after division by n is considered. For example, let n = 7. Then, in modular arithmetic modulo 7, the sum 3 + 5 is 1 instead of 8, since 8 divided by 7 has remainder 1. Similarly, 6 + 1 = 0 modulo 7, 2 − 5 = 4 modulo 7 (since −3 + 7 = 4) and 3 · 4 = 5 modulo 7 (12 has remainder 5). Standard properties of addition and multiplication familiar from the number system of the integers or rational numbers remain valid, for example
- (a + b) · c = a · c + b · c (law of distributivity).
In general it is, however, not possible to divide in this setting. For example, for n = 6, the equation
- 3 · x = 2 (modulo 6),
a solution x of which would be an analogue of 2/3, cannot be solved, as one can see by calculating 3 · 0, ..., 3 · 5 modulo 6. The distinctive feature of prime numbers is the following: division is possible in modular arithmetic if and only if n is a prime. For n = 7, the equation
- 3 · x = 2 (modulo 7)
has a unique solution, x = 3. Equivalently, n is prime if and only if all integers m satisfying 2 ≤ m ≤ n − 1 are coprime to n, i.e., their greatest common divisor is 1. Using Euler's totient function φ, n is prime if and only if φ(n) = n − 1.
The set {0, 1, 2, ..., n − 1}, with addition and multiplication is denoted Z/nZ for all n. In the parlance of abstract algebra, it is a ring, for any n, but a finite field if and only if n is prime. A number of theorems can be derived from inspecting Z/pZ in an abstract way. For example Fermat's little theorem, stating that ap − a is divisible by p for any integer a, may be proved using these notions. A consequence of this is the following: if p is a prime number other than 2 and 5, 1/p is always a recurring decimal, whose period is p − 1 or a divisor of p − 1. The fraction 1/p expressed likewise in base q (rather than base 10) has similar effect, provided that p is not a prime factor of q. Wilson's theorem says that an integer p > 1 is prime if and only if the factorial (p − 1)! + 1 is divisible by p. Moreover, an integer n > 4 is composite if and only if (n − 1)! is divisible by n.
[edit]Other mathematical occurrences of primes
Many mathematical domains make great use of prime numbers. An example from the theory of finite groups are the Sylow theorems: if G is a finite group and pn is the highest power of the prime p which divides the order of G, then G has a subgroup of order pn. Also, any group of prime order is cyclic (Lagrange's theorem).
[edit]Public-key cryptography
Main article: Public key cryptography
Several public-key cryptography algorithms, such as RSA and the Diffie–Hellman key exchange, are based on large prime numbers (for example 512 bit primes are frequently used for RSA and 1024 bit primes are typical for Diffie–Hellman.). RSA relies on the fact that it is thought to be much easier (i.e., more efficient) to perform the multiplication of two (large) numbers x and y than to calculate x and y (assumed coprime) if only the product xy is known. The Diffie–Hellman key exchange relies on the fact that there are efficient algorithms for modular exponentiation, while the reverse operation the discrete logarithm is thought to be a hard problem.
[edit]Prime numbers in nature
Inevitably, some of the numbers that occur in nature are prime. There are, however, relatively few examples of numbers that appear in nature because they are prime.
One example of the use of prime numbers in nature is as an evolutionary strategy used by cicadas of the genus Magicicada.[30] These insects spend most of their lives as grubs underground. They only pupate and then emerge from their burrows after 13 or 17 years, at which point they fly about, breed, and then die after a few weeks at most. The logic for this is believed to be that the prime number intervals between emergences make it very difficult for predators to evolve that could specialise as predators onMagicicadas.[31] If Magicicadas appeared at a non-prime number intervals, say every 12 years, then predators appearing every 2, 3, 4, 6, or 12 years would be sure to meet them. Over a 200-year period, average predator populations during hypothetical outbreaks of 14- and 15-year cicadas would be up to 2% higher than during outbreaks of 13- and 17-year cicadas.[32] Though small, this advantage appears to have been enough to drive natural selection in favour of a prime-numbered life-cycle for these insects.
There is speculation that the zeros of the zeta function are connected to the energy levels of complex quantum systems.[33]
[edit]Generalizations
The concept of prime number is so important that it has been generalized in different ways in various branches of mathematics. Generally, "prime" indicates minimality or indecomposability, in an appropriate sense. For example, the prime field is the smallest subfield of a field F containing both 0 and 1. It is either Q or the finite field with p elements, whence the name. Often a second, additional meaning is intended by using the word prime, namely that any object can be, essentially uniquely, decomposed into its prime components. For example, in knot theory, a prime knot is a knot which is indecomposable in the sense that it cannot be written as the knot sum of two nontrivial knots. Any knot can be uniquely expressed as a connected sum of prime knots.[34] Prime models and prime 3-manifolds are other examples of this type.
[edit]Prime elements in rings
Main articles: Prime element and Irreducible element
Prime numbers give rise to two more general concepts that apply to elements of any ring R, an algebraic structure where addition, subtraction and multiplication are defined: prime elements and irreducible elements. An element p of R is called prime if it is not a unit (i.e., does not have a multiplicative inverse) and the following property holds: given x and y in R such that p divides the product, then p divides at least one factor. Irreducible elements are ones which cannot be written as a product of two ring elements that are not units. In general, this is a weaker condition, but for any unique factorization domain, such as the ring Z of integers, the set of prime elements equals the set of irreducible elements, which for Z is :
- {…, −11, −7, −5, −3, −2, 2, 3, 5, 7, 11, …}.
A common example is the Gaussian integers Z[i], that is, the set of complex numbers of the form a + bi with a and b in Z. This is an integral domain, its prime elements are known as Gaussian primes. Not every prime (in Z) is a Gaussian prime: in the bigger ring Z[i], 2 factors into the product of the two Gaussian primes (1 + i) and (1 − i). Rational primes (i.e. prime elements in Z) of the form 4k + 3 are Gaussian primes, whereas rational primes of the form 4k + 1 are not. Gaussian primes can be used in proving quadratic reciprocity, while Eisenstein primes play a similar role for cubic reciprocity.
[edit]Prime ideals
Main article: Prime ideals
In ring theory, the notion of number is generally replaced with that of ideal. Prime ideals, which generalize prime elements in the sense that the principal ideal generated by a prime element is a prime ideal, are an important tool and object of study incommutative algebra, algebraic number theory and algebraic geometry. The prime ideals of the ring of integers are the ideals (0), (2), (3), (5), (7), (11), … The fundamental theorem of arithmetic generalizes to the Lasker–Noether theorem which expresses any ideal in a Noetherian commutative ring as the intersection of primary ideals, which are the appropriate generalizations of prime powers.[35]
Prime ideals are the points of algebro-geometric objects, via the notion of the spectrum of a ring. Arithmetic geometry also benefits from this notion, and many concepts exist in both geometry and number theory. For example, factorization or ramification of prime ideals when lifted to an extension field, a basic problem of algebraic number theory, bears some resemblance with ramification in geometry.
[edit]Primes in valuation theory
In algebraic number theory, yet another generalization is used. A starting point for valuation theory is the p-adic valuations, where p is a prime number. It tells what highest power p divides a given number n. Using that, the p-adic norm is set up, which, in contrast to the usual absolute value, gets smaller when a number is multiplied by p. The completion of Q (the field of rational numbers) with respect to this norm leads to Qp, the field of p-adic numbers, as opposed to R, the reals, which are the completion with respect to the usual absolute value. To highlight the connection to primes, the absolute value is often called the infinite prime. These are essentially all possible ways to complete Q, by Ostrowski's theorem.
In an arbitrary field K, one considers valuations on K, certain functions from K to the real numbers R. Every such valuation yields a topology on K, and two valuations are called equivalent if they yield the same topology. A prime of K (sometimes called aplace of K) is an equivalence class of valuations.
Arithmetic questions related to, global fields such as Q may, in certain cases, be transferred back and forth to the completed fields (known as local fields), a concept known as local-global principle. This again underlines the importance of primes to number theory.
[edit]In the arts and literature
Prime numbers have influenced many artists and writers. The French composer Olivier Messiaen used prime numbers to create ametrical music through "natural phenomena". In works such as La Nativité du Seigneur (1935) and Quatre études de rythme(1949–50), he simultaneously employs motifs with lengths given by different prime numbers to create unpredictable rhythms: the primes 41, 43, 47 and 53 appear in one of the études. According to Messiaen this way of composing was "inspired by the movements of nature, movements of free and unequal durations".[36]
In his science fiction novel Contact, later made into a film of the same name, the NASA scientist Carl Sagan suggested that prime numbers could be used as a means of communicating with aliens, an idea that he had first developed informally with American astronomer Frank Drake in 1975.[37]
Many films reflect a popular fascination with the mysteries of prime numbers and cryptography: films such as Cube, Sneakers, The Mirror Has Two Faces and A Beautiful Mind, the latter of which is based on the biography of the mathematician and Nobel laureate John Forbes Nash by Sylvia Nasar.[38] Prime numbers are used as a metaphor for loneliness and isolation in the Paolo Giordano novel The Solitude of Prime Numbers, in which they are portrayed as "outsiders" among integers.[39]
[edit]See also
- Bonse's inequality
- Brun's constant
- Burnside theorem
- Chebotarev's density theorem
- Chen's theorem
- Chinese remainder theorem
- Classification of finite simple groups
- Copeland–Erdős constant
- Eisenstein's criterion
- Illegal prime
- Integer factorization
- Large sieve
- List of prime numbers
- Mathematics in medieval Islam
- Multiplicative function
- Multiplicative number theory
- Paillier cryptosystem
- Prime power
- Primon gas
- Quadratic residuosity problem
- Ramanujan–Petersson conjecture
- Regular prime
[edit]Distributed computing projects that search for primes
- PrimeGrid searches for megaprimes.
- Wieferich@Home searches for Wieferich primes.
- GIMPS searches for Mersenne primes.
[edit]Notes
- ^ (sequence A000040 in OEIS).
- ^ "Euclid's Elements, Book IX, Proposition 20". Aleph0.clarku.edu. Retrieved 2010-08-23.
- ^ GIMPS Home; http://www.mersenne.org/
- ^ Riesel 1994, p. 36
- ^ Conway & Guy 1996, pp. 129–130
- ^ Derbyshire, John (2003), "The Prime Number Theorem", Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics, Washington, D.C.: Joseph Henry Press, p. 33, ISBN 9780309085496, OCLC 249210614
- ^ Gowers 2002, p. 118 "The seemingly arbitrary exclusion of 1 from the definition of a prime … does not express some deep fact about numbers: it just happens to be a useful convention, adopted so there is only one way of factorizing any given number into primes."
- ^ ""Why is the number one not prime?"". Retrieved 2007-10-02.
- ^ ""Arguments for and against the primality of 1".
- ^ The Largest Known Prime by Year: A Brief History Prime Curios!: 17014…05727 (39-digits)
- ^ Hardy 1908, pp. 122–123
- ^ Letter in Latin from Goldbach to Euler, July 1730.
- ^ Ribenboim 2004, p. 4
- ^ Füstenberg 1955
- ^ (Ben Green & Terence Tao 2008).
- ^ (Lehmer 1909).
- ^ Chris K. Caldwell. "The Top Twenty: Factorial". Primes.utm.edu. Retrieved 2010-10-07.
- ^ Chris K. Caldwell. "The Top Twenty: Primorial". Primes.utm.edu. Retrieved 2010-08-23.
- ^ http://primes.utm.edu/top20/page.php?id=1
- ^ "Record 12-Million-Digit Prime Number Nets $100,000 Prize". Electronic Frontier Foundation. October 14, 2009. Retrieved 2010-01-04.
- ^ "EFF Cooperative Computing Awards". Electronic Frontier Foundation. Retrieved 2010-01-04.
- ^ Havil 2003, p. 163
- ^ Havil 2003, p. 171
- ^ See list of values, calculated by Wolfram Alpha
- ^ Hua (2009), p. 176-177"
- ^ Caldwell, Chris, The Top Twenty: Lucas Number at The Prime Pages.
- ^ E.g., see Guy 1981, problem A3, pp. 7–8
- ^ Weisstein, Eric W., "Landau's Problems" from MathWorld.
- ^ Hardy 1940 "No one has yet discovered any warlike purpose to be served by the theory of numbers or relativity, and it seems unlikely that anyone will do so for many years."
- ^ Goles, E., Schulz, O. and M. Markus (2001). "Prime number selection of cycles in a predator-prey model", Complexity 6(4): 33-38
- ^ Paulo R. A. Campos, Viviane M. de Oliveira, Ronaldo Giro, and Douglas S. Galvão. (2004), "Emergence of Prime Numbers as the Result of Evolutionary Strategy", Phys. Rev. Lett. 93: 098107, doi:10.1103/PhysRevLett.93.098107, retrieved 2006-11-26.
- ^ "Invasion of the Brood". The Economist. May 6, 2004. Retrieved 2006-11-26.
- ^ Ivars Peterson (June 28, 1999). "The Return of Zeta". MAA Online. Retrieved 2008-03-14.
- ^ Schubert, H. "Die eindeutige Zerlegbarkeit eines Knotens in Primknoten". S.-B Heidelberger Akad. Wiss. Math.-Nat. Kl. 1949 (1949), 57–104.
- ^ Eisenbud 1995, section 3.3.
- ^ Hill, ed. 1995
- ^ Carl Pomerance, Prime Numbers and the Search for Extraterrestrial Intelligence, Retrieved on December 22, 2007
- ^ The music of primes, Marcus du Sautoy's selection of films featuring prime numbers.
- ^ "Introducing Paolo Giordano". Books Quarterly.
[edit]References
- Apostol, Thomas M. (1976), Introduction to Analytic Number Theory, New York: Springer, ISBN 0-387-90163-9
- Conway, John Horton; Guy, Richard K. (1996), The Book of Numbers, New York: Copernicus, ISBN 978-0-387-97993-9
- Crandall, Richard; Pomerance, Carl (2005), Prime Numbers: A Computational Perspective (2nd ed.), Berlin, New York: Springer-Verlag, ISBN 978-0-387-25282-7
- Derbyshire, John (2003), Prime obsession, Joseph Henry Press, Washington, DC, MR1968857, ISBN 978-0-309-08549-6
- Eisenbud, David (1995), Commutative algebra, Graduate Texts in Mathematics, 150, Berlin, New York: Springer-Verlag, MR1322960, ISBN 978-0-387-94268-1
- Furstenberg, Harry (1955), "On the infinitude of primes", The American Mathematical Monthly (Mathematical Association of America) 62 (5): 353, doi:10.2307/2307043, ISSN 0002-9890
- Green, Ben; Tao, Terence (2008), "The primes contain arbitrarily long arithmetic progressions", Annals of Mathematics 167: 481–547, doi:10.4007/annals.2008.167.481, arXiv:math.NT/0404188
- Gowers, Timothy (2002), Mathematics: A Very Short Introduction, Oxford University Press, ISBN 978-0-19-285361-5
- Guy, Richard K. (1981), Unsolved Problems in Number Theory, Berlin, New York: Springer-Verlag, ISBN 978-0-387-90593-8
- Havil, Julian (2003), Gamma: Exploring Euler's Constant, Princeton University Press, ISBN 978-0-691-09983-5
- Hardy, Godfrey Harold (1908), A Course of Pure Mathematics, Cambridge University Press, ISBN 978-0-521-09227-2
- Hardy, Godfrey Harold (1940), A Mathematician's Apology, Cambridge University Press, ISBN 978-0-521-42706-7
- Lehmer, D. H. (1909), Factor table for the first ten millions containing the smallest factor of every number not divisible by 2, 3, 5, or 7 between the limits 0 and 10017000, Washington, D.C.: Carnegie Institution of Washington
- Narkiewicz, Wladyslaw (2000), The development of prime number theory: from Euclid to Hardy and Littlewood, Springer Monographs in Mathematics, Berlin, New York: Springer-Verlag, ISBN 978-3-540-66289-1
- Ribenboim, Paulo (2004), The little book of bigger primes, Berlin, New York: Springer-Verlag, ISBN 978-0-387-20169-6
- Riesel, Hans (1994), Prime numbers and computer methods for factorization, Basel, Switzerland: Birkhäuser, ISBN 978-0-8176-3743-9
- Sabbagh, Karl (2003), The Riemann hypothesis, Farrar, Straus and Giroux, New York, MR1979664, ISBN 978-0-374-25007-2
- du Sautoy, Marcus (2003), The Music of Primes website The music of the primes, HarperCollins Publishers, MR2060134, ISBN 978-0-06-621070-4
- Hua, L. K. (2009). Additive Theory of Prime Numbers. Translations of Mathematical Monographs. 13. AMS Bookstore. ISBN 978-0-8218-4942-2.
[edit]Further references
- Hill, Peter Jensen, ed. (1995), The Messiaen companion, Portland, Or: Amadeus Press, ISBN 978-0-931340-95-6
- Kelly, Katherine E., ed. (2001), The Cambridge companion to Tom Stoppard, Cambridge University Press, ISBN 978-0-521-64592-8
- Stoppard, Tom (1993), Arcadia, London: Faber and Faber, ISBN 978-0-571-16934-4
[edit]External links
| Wikinews has related news:Two largest known prime numbers discovered just two weeks apart, one qualifies for $100k prize |
- Caldwell, Chris, The Prime Pages at primes.utm.edu.
- Prime Numbers at MathWorld
- MacTutor history of prime numbers
- The prime puzzles
- An English translation of Euclid's proof that there are infinitely many primes
- Number Spiral with prime patterns
- An Introduction to Analytic Number Theory, by Ilan Vardi and Cyril Banderier
- EFF Cooperative Computing Awards
- Why a Number Is Prime by Enrique Zeleny, Wolfram Demonstrations Project.
- Plus teacher and student package: prime numbers from Plus, the free online mathematics magazine produced by the Millennium Mathematics Project at the University of Cambridge
- Prime Numbers Spirals and Visual Patterns by Luis Mateos
[edit]Prime number generators and calculators
- Online Prime Number Generator and Checker - instantly checks and finds prime numbers up to 128 digits long (does NOT require Java or JavaScript)
- Prime Number Checker identifies the smallest prime factor of a number
- Fast Online primality test — Dario Alpern's personal site – Makes use of the Elliptic Curve Method (up to thousands digits numbers check!, requires Java)
- Prime Number Generator — Generates a given number of primes above a given start number.
- Primes from WIMS is an online prime generator.
- Huge database of prime numbers
- All prime numbers below 10,000,000,000
Prime power
From Wikipedia, the free encyclopedia
For the electrical generator power rating, see Prime power (electrical).
In mathematics, a prime power is a positive integer power of a prime number. For example: 5=51, 9=32 and 16=24 are prime powers, while 6=2×3, 15=3×5 and 36=62=22×32 are not. The twenty smallest prime powers are (sequence A000961 in OEIS):
The prime powers are those positive integers that are divisible by just one prime number; prime powers and related concepts are also called primary numbers, as in the primary decomposition.
Contents[hide] |
[edit]Properties
[edit]Algebraic properties
Every prime power (except powers of 2) has a primitive root; thus the multiplicative group of integers modulo pn (or equivalently, the unit group of the ring  ) is cyclic.
) is cyclic.
 ) is cyclic.
) is cyclic.
The number of elements of a finite field is always a prime power and conversely, every prime power occurs as the number of elements in some finite field (which is unique up to isomorphism).
[edit]Combinatorial properties
A property of prime powers used frequently in analytic number theory is that the set of prime powers which are not prime is a small set in the sense that the infinite sum of their reciprocals converges, although the primes are a large set.
[edit]Divisibility properties
The totient function (φ) and sigma functions (σ0) and (σ1) of a prime power are calculated by the formulas:
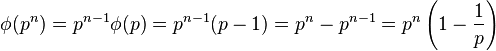 ,
,
 ,
,
 .
.
All prime powers are deficient numbers. A prime power pn is an n-almost prime. It is not known whether a prime power pn can be an amicable number. If there is such a number, then pn must be greater than 101500 and n must be greater than 1400.
[edit]Popular media
In the 1997 film Cube, prime powers play a key role, acting as indicators of lethal dangers in a maze-like cube structure.
[edit]See also
[edit]References
- Elementary Number Theory. Jones, Gareth A. and Jones, J. Mary. Springer-Verlag London Limited. 1998.
leave your comments lets share the claymath 1 million dollar prize, as far as i can see by intuition there must be a relation between
Best Regards and Merry Xmas and Happy New Year 2011.
Yours Sincerely DMSP
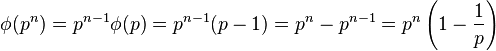
Propiedades básicas
[editar]Algunos valores
- Algunos valores exactos
Euler fue capaz de encontrar una fórmula cerrada para ζ(2k) cuando k es un entero positivo:
donde B2k son los números de Bernoulli. De esta fórmula se obtiene que: ζ(2) = π²/6, ζ(4) = π4/90, ζ(6) = π6/945 etc. Para números impares no se conoce una solución general.
Para valores negativos, si k ≥ 1, entonces
Se puede ver que para los números pares negativos, la función zeta de Riemann se anula, denominándose éstos como ceros triviales.

 corresponde a la serie armónica.
corresponde a la serie armónica.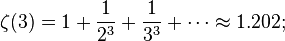 es la constante de Apéry.
es la constante de Apéry.
[editar]Ecuación funcional
La función zeta de Riemann se puede prolongar analíticamente para todo número complejo excepto s=1, mediante la siguiente ecuación funcional:
La ecuación tiene un polo simple en s=1 con residuo 1 y fue demostrada por Bernhard Riemann en 1859 en su ensayo Sobre el número de números primos menores que una cantidad dada. Una relación equivalente fue conjeturizada por Euler para la función  .
.
 .
.
También hay una versión simétrica de la ecuación funcional bajo el cambio  .
.
 .
.
donde Γ(s) es la función gamma.
En algunas ocasiones se define la función:
con lo que
La ecuación funcional también cumple el siguiente límite asintótico:
[editar]Ceros de la función
El valor de la función zeta para los números pares negativos es 0 (viendo la ecuación funcional es evidente), por lo que son llamados ceros triviales. Aparte de los ceros triviales, la función también se anula en valores de s que están dentro del rango {s ∈ C: 0 < Re(s) < 1}, y que son llamados ceros no triviales, debido a que es más difícil demostrar la ubicación de esos ceros dentro del rango crítico. El estudio de la distribución de estos «ceros no triviales» es muy importante, debido a que tiene profundas implicaciones en la distribución de los números primos y en cuestiones relacionadas con la teoría de números. La hipótesis de Riemann, considerado uno de los mayores problemas matemáticos abiertos en la actualidad, asegura que cualquier cero no trivial tiene que Re(s)=1/2, por lo tanto, todos los ceros están alineados en el plano complejo formando una recta, llamadarecta crítica.
La localización de estos ceros tiene significativa importancia en teoría de números, ya que, por ejemplo, el hecho de que todos los ceros estén en el rango crítico demuestra elteorema de los números primos. Un mejor resultado es que ζ(σ + it) ≠ 0 para cualquier |t| ≥ 3 y
También es conocido que existen infinitos ceros sobre la recta crítica, como mostró G.H. Hardy y Littlewood.
[editar]Recíproco de la función
El reciproco de la función zeta puede ser expresado mediante una serie de Dirichlet sobre la función de Möbius μ(n) , definido para cualquier número complejo s con la parte real mayor que 1 como:
existen otras expresiones de este tipo que hacen uso de funciones multiplicativas como puede ser
donde φ(n) es la función φ de Euler.
[editar]Universalidad
La función zeta tiene la notable propiedad de universalidad. Esta universalidad dice que existe alguna localización dentro del rango crítico que se aproxima a cualquier función holomorfa bastante bien. Como este tipo de funciones es bastante general, esta propiedad es bastante importante.
[editar]Representaciones
La función zeta de Riemann tiene distintas representaciones, siendo algunas las que se muestran a continuación:
[editar]Transformada de Mellin
- Para valores de s con la parte real mayor que uno se tiene que
- La transformada de Mellin de la función 1/(exp(x)-1) es precisamente la expresión anterior. O sea:
- También se puede relacionar con los números primos y el teorema de los números primos. Si π(x) es la función contador de números primos, entonces:
- convergente para valores Re(s)>1. Si se define la función ω(s) como
- entonces la transformada de Mellin
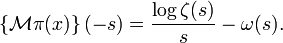
- Una tranformada de Mellin similar, que relaciona la función contador de primos de Riemann, definida como
 es:
es:
[editar]Series de Laurent
La función zeta es meromorfa con un polo simple en s=1. Ésta puede expandirse como una serie de Laurent en torno a s=1, la serie resultante es:
donde las constantes γn, son llamadas constantes de Stieltjes y son definidas como:
La constante γ0 corresponde a la constante de Euler-Mascheroni.
[editar]Producto de Hadamard
Utilizando el teorema de factorización de Weierstrass, Hadamard dio una expansión en forma de producto infinito de la función zeta:
donde el producto es sobre todos los ceros no triviales ρ de ζ y la letra γ corresponde a la constante de Euler-Mascheroni. Una forma más simple es:
De esta forma elegante se puede observar el polo simple en s=1 (denominador), los ceros triviales dados por el término de la función gamma (denominador), y los ceros no triviales, dados cuando s=ρ (numerador).
[editar]Serie global
Una representación en forma de serie, convergente para todo número complejo s, excepto 1, fue conjeturizada por Konrad Knopp y probada por Helmut Hasse en 1930:
[editar]Aplicaciones
Aunque los matemáticos consideran que la función zeta tiene un interés principal en la «más pura» de las disciplinas matemáticas, la teoría de números, lo cierto es que también tiene aplicaciones en estadística y en física. En algunos cálculos realizados en física, se debe evaluar la suma de los números enteros positivos. Paradójicamente, por motivos físicos se espera una respuesta finita. Cuando se produce esta situación, hay normalmente un enfoque riguroso con un análisis en profundidad, así como un «atajo», usando la función zeta de Riemann. El argumento es el siguiente:
- Queremos evaluar la suma 1 + 2 + 3 + 4 + ... , pero podemos reescribirlo como una suma de sus inversos.
La suma S parece tomar la forma de 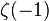 . Sin embargo, −1 sale fuera del dominio de convergencia de la serie de Dirichlet para la función zeta. Sin embargo, una serie divergente con términos positivos como ésta a veces puede ser representada de forma razonable por el método de sumación de Ramanujan. Este método de suma implica la aplicación de la fórmula de Euler-Maclaurin, y cuando se aplica a la función zeta, su definición se extiende a todo el plano complejo. En particular,
. Sin embargo, −1 sale fuera del dominio de convergencia de la serie de Dirichlet para la función zeta. Sin embargo, una serie divergente con términos positivos como ésta a veces puede ser representada de forma razonable por el método de sumación de Ramanujan. Este método de suma implica la aplicación de la fórmula de Euler-Maclaurin, y cuando se aplica a la función zeta, su definición se extiende a todo el plano complejo. En particular,
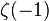 . Sin embargo, −1 sale fuera del dominio de convergencia de la serie de Dirichlet para la función zeta. Sin embargo, una serie divergente con términos positivos como ésta a veces puede ser representada de forma razonable por el método de sumación de Ramanujan. Este método de suma implica la aplicación de la fórmula de Euler-Maclaurin, y cuando se aplica a la función zeta, su definición se extiende a todo el plano complejo. En particular,
. Sin embargo, −1 sale fuera del dominio de convergencia de la serie de Dirichlet para la función zeta. Sin embargo, una serie divergente con términos positivos como ésta a veces puede ser representada de forma razonable por el método de sumación de Ramanujan. Este método de suma implica la aplicación de la fórmula de Euler-Maclaurin, y cuando se aplica a la función zeta, su definición se extiende a todo el plano complejo. En particular,
donde la notación  indica suma de Ramanujan. Para exponentes pares se tiene que:
indica suma de Ramanujan. Para exponentes pares se tiene que:
 indica suma de Ramanujan. Para exponentes pares se tiene que:
indica suma de Ramanujan. Para exponentes pares se tiene que:
y para exponentes impares, se obtiene la relación con los números de Bernoulli:
La regularización de la función zeta se utiliza como un posible medio de la regularización de series divergentes en teoría cuántica de campos. Como ejemplo notable, la función zeta de Riemann aparece explícitamente en el cálculo del efecto Casimir.
Best Regards and Merry Xmas and Happy New Year 2011.
Yours Sincerely DMSP
Hipótesis de Riemann
En matemática pura, la hipótesis de Riemann, formulada por primera vez por Bernhard Riemann en 1859, es una conjetura sobre la distribución de los ceros de la función zeta de Riemann ζ(s).
La hipótesis de Riemann, por su relación con la distribución de los números primos en el conjunto de los naturales, es uno de los problemas abiertos más importantes en la matemática contemporánea.
Se ha ofrecido un premio de un millón de dólares por el Instituto Clay de Matemáticas para la primera persona que desarrolle una demostración correcta de la conjetura. La mayoría de la comunidad matemática piensa que la conjetura es cierta, aunque otros grandes matemáticos como J. E. Littlewood y Atle Selberg se mostraron escépticos, si bien el escepticismo de Selberg fue disminuyendo desde sus días de juventud. En un artículo en 1989 sugirió que un análogo debe ser cierto para una clase mucho más amplia de funciones (la clase de Selberg).
Contenido[ocultar] |
[editar]Definición
La función zeta de Riemann ζ(s) está definida de la siguiente manera:
Para todos los números complejos s ≠ 1, se puede prolongar analíticamente mediante la ecuación funcional:

Ésta posee ciertos valores, llamados ceros "triviales" para los cuales la función zeta se anula. De la ecuación se puede ver que s = −2, s = −4, s = −6, ... son ceros triviales. Existen otros valores complejos s comprendidos entre 0 < Re(s) < 1, para los cuales la función zeta también se anula, llamados ceros "no triviales". La conjetura de Riemann hace referencia a éstos ceros no triviales afirmando:
|
Por lo tanto los ceros no triviales deberían encontrarse en la línea crítica s = 1/2 + i t donde t es un número real e i es la unidad imaginaria. La función zeta de Riemann, a lo largo de la línea crítica ha sido estudiada en términos de la función Z, cuyos ceros corresponden a los ceros de la función zeta sobre la línea crítica.
[editar]Historia
Riemann mencionó la conjetura, que sería llamada la hipótesis de Riemann, en su artículo de 1859 Sobre los números primos menores que una magnitud dada, al desarrollar una fórmula explícita para calcular la cantidad de primos menores que x. Puesto que no era esencial para el propósito central de su artículo, no intentó dar una demostración de la misma. Riemann sabía que los ceros no triviales de la función zeta están distribuidos en torno a la recta s = 1/2 + i t, y sabía también que todos los ceros no triviales debían estar en el rango 0 ≤ Re(s) ≤ 1.1
En 1896, Hadamard y de la Vallée-Poussin probaron independientemente, que ningún cero podía estar sobre la recta Re(s) = 1. Junto con las otras propiedades de los ceros no triviales demostradas por Riemann, esto mostró que todos los ceros no triviales deben estar en el interior de la banda crítica 0 < Re(s) < 1. Este fue un paso fundamental para las primeras demostraciones del teorema de los números primos.
En 1900, Hilbert incluyó la hipótesis de Riemann en su famosa lista de los 23 problemas no resueltos — es parte del problema 8 en la lista de Hilbert junto con la conjetura de Goldbach. Cuando se le preguntó qué haría si se despertara habiendo dormido quinientos años, remarcablemente Hilbert contestó que su primera pregunta sería si la hipótesis de Riemann había sido probada. La hipótesis de Riemann es el único problema de los que propuso Hilbert que está en el premio del milenio del Instituto Clay de Matemáticas.
En 1914, Hardy demostró que existe un número infinito de ceros sobre la recta crítica Re(s) = 1/2. Sin embargo todavía era posible que un número infinito (y posiblemente la mayoría) de los ceros no triviales se encontraran en algún otro lugar sobre la banda crítica. En trabajos posteriores de Hardy y Littlewood en 1921 y de Selberg en 1942 se dieron estimaciones para la densidad promedio de los ceros sobre la línea crítica.
Trabajos recientes se han concentrado en el cálculo explícito de la localización de grandes cantidades de ceros (con la esperanza de hallar algún contraejemplo) y en el establecimientos de cotas superiores en la proporción de ceros que puedan estar lejos de la línea crítica (con la esperanza de reducirlas a cero).
[editar]La hipótesis de Riemann y los números primos
La formulación tradicional de la hipótesis de Riemann oscurece un poco la importancia real de la conjetura. La función zeta de Riemann tiene una profunda conexión con los números primos y Hege von Koch demostró en 1901 que la hipótesis de Riemann es equivalente al considerable refinamiento del teorema de los números primos: Existe una constante C > 0 tal que

para todo x suficientemente grande, donde π(x) es la función contadora de primos y ln(x) es el logaritmo natural de x. Lowell Schoenfeld mostró que se puede tomar C = 1/(8 π) para todo x ≥ 2657.
Los ceros de la función zeta y los números primos satisfacen ciertas propiedades de dualidad, conocidas como fórmulas explícitas, que muestran, usando análisis de Fourier, que los ceros de la función zeta de Riemann pueden interpretarse como frecuencias armónicas en la distribución de los números primos.
Más aún, si la conjetura de Hilbert-Polya es cierta, entonces cualquier operador que nos dé las partes imaginarias de los ceros como sus valores propios debe satisfacer:
donde Tr es la traza del operador (suma de sus valores propios) , β es un número imaginario y ψ(x) es la Función de Chebyshov que nos suma el log(x) sobre los primos y sus potencias enteras, dicha fórmula es una conclusion de la 'fórmula explicita' de V. Mangoldt.2 Varios operadores propuestos por C. Perelman, J. Macheca y J. Garcia, parecen corroborar los resultados de la conjetura de Hilbert sobre el operador, reproduciendo la parte imaginaria de los ceros. Usando la teoria WKB o de Cuantizacion de Bohr-Sommerfeld uno puede probar que si el potencial V(x) de un Hamiltoniano H cuyas energias sean las partes imaginarias de los ceros no triviales debe cumplir (ecuacion implicita)  donde N(T) es el numero de ceros con parte imaginaria menor que un dado T >0 y
donde N(T) es el numero de ceros con parte imaginaria menor que un dado T >0 y  significa la derivada fraccional de orden 1/2
significa la derivada fraccional de orden 1/2
 donde N(T) es el numero de ceros con parte imaginaria menor que un dado T >0 y
donde N(T) es el numero de ceros con parte imaginaria menor que un dado T >0 y  significa la derivada fraccional de orden 1/2
significa la derivada fraccional de orden 1/2[editar]Cálculo numérico
- En el año 2004 Xavier Gourdon verificó la conjetura de Riemann numéricamente a lo largo de los primeros diez trillones de ceros no triviales de la función. Sin embargo esto no es estrictamente una demostración, numéricamente es más interesante encontrar un contraejemplo, es decir un valor de cero que no cumpla con que su parte real es 1/2, pues esto echaría por los suelos la validez de la conjetura.
- Hasta el 2005, el intento más serio para explorar los ceros de la función-ζ, es el ZetaGrid, un proyecto de computación distribuida con la capacidad de verificar billones de ceros por día. El proyecto acabó en diciembre de 2005,y ninguno de los ceros pudo ser identificado como contraejemplo de la hipótesis de Riemann.
[editar]Véase también
- Teorema de los números primos
- Función zeta de Riemann
- Función contador de números primos
- Fórmula explícita
- Teoría analítica de números
[editar]Referencias
- ↑ Riemann, Bertrand (1859). «Ueber die Anzahl der Primzahlen unter einer gegebenen Grösse». Consultado el 29 de diciembre de 2008.
- ↑ Explicit formula http://www.wbabin.net/science/moreta8.pdf
[editar]Enlaces externos
- (en) A proof of the Riemann hypothesis
- (en) The Riemann Hypothesis in a Nutshell
- (en) Andrew Odlyzko: Tables of zeros of the Riemann zeta function
- (en) Zetagrid
- (en) Algoritmos para calcular los ceros de la función de Riemann, por Michael Rubinstein
- (es) Determinación geométrica de los números primos y perfectos
- (en) La funcion Zeta de Riemann en la linea critica como un determinante funcional
The First 10,000 Primes
(the 10,000th is 104,729)
For more information on primes see http://primes.utm.edu/
2 3 5 7 11 13 17 19 23 29
31 37 41 43 47 53 59 61 67 71
73 79 83 89 97 101 103 107 109 113
127 131 137 139 149 151 157 163 167 173
179 181 191 193 197 199 211 223 227 229
233 239 241 251 257 263 269 271 277 281
283 293 307 311 313 317 331 337 347 349
353 359 367 373 379 383 389 397 401 409
419 421 431 433 439 443 449 457 461 463
467 479 487 491 499 503 509 521 523 541
547 557 563 569 571 577 587 593 599 601
607 613 617 619 631 641 643 647 653 659
661 673 677 683 691 701 709 719 727 733
739 743 751 757 761 769 773 787 797 809
811 821 823 827 829 839 853 857 859 863
877 881 883 887 907 911 919 929 937 941
104179 104183 104207 104231 104233 104239 104243 104281 104287 104297
104309 104311 104323 104327 104347 104369 104381 104383 104393 104399
104417 104459 104471 104473 104479 104491 104513 104527 104537 104543
104549 104551 104561 104579 104593 104597 104623 104639 104651 104659
104677 104681 104683 104693 104701 104707 104711 104717 104723 104729
i will propose a new mathematical algorithm based on rsa encoding but with matrixes
the parameters for a written text we should to send are number of rows in wich is divided the matrix one number is equivalent to one complete stand alone word, the number of columns of the matrix
the system verifies that A (source nonencripted word matrix) * (B (encripted matrix) ) = (E+H) (private key diagonal not prime numbers matrix with high values the higher they are the difficlut to unencrypt) I in which C=D+E,F=G+H witch are two matrixes that are also diagonal and are composed by primes and satisfies conjecture of goldback , D=private key , E = public key,F,G private keys H public key same correspondence and satisfaction than C=D+E
A=B^-1=A^t/|A|=>Aij*Aij^t/|A|=
so now we can obtained both encoding for encrypted and not encrypted text as well as public/private keys once defined C and F
thank you very much and best regards



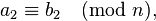



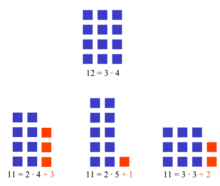

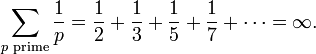






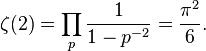


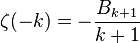





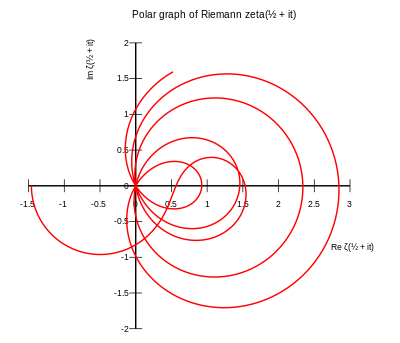






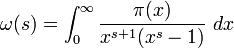



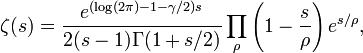






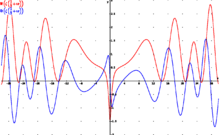


![\sum_{n}e^{-\beta E_{n}}=Tr[e^{-\beta \hat H}]=e^{u/2}-e^{-u/2} \frac{d\psi _{0}}{du}-\frac{e^{u/2}}{e^{3u}-e^{u}},](http://upload.wikimedia.org/math/0/8/4/084a91626171144d3b39500d69487a8a.png)
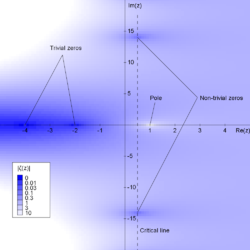
chequeandoo nuevas entradas...
ResponderEliminarEste comentario ha sido eliminado por el autor.
ResponderEliminara true story , once a phisician went to a famous waterfall electric generator, the generator was broken it has a derivation of the current flux the phisician turn on make measurments of the magnetic field turn off and spent two days making complex calculations,within the two days he had to spent sleeping one night at the generator after the two days he just cut two wires of the reel and join them with aislant tape, he went to the boss of the plant and said:
ResponderEliminarI concluded my work, try it .
the generator again worked at its maximum power.
How much is it? said the boss of the plant.
100,000 dollars
100,000 dollars? thats expensive for a wire join.
no, i beg you 1 dollar for joining with the tap and 99,999 for the calculations i have to made.
never misconsider others thoughts due thinking its also can be an expensive job. but after all we are human people and to be a good person with the people that surrounds you to make their lives easier it is more important than the complex of the thoughts.
David